解构巴赫 DeBach
—巴赫《平均律钢琴曲集》视觉化研究/The Visualization of Bach’s “Well-Tempered Clavichord”硕士学位毕业设计/M.F.A. Thesis
RIVES BFK 低克数版画纸,聚氯乙烯片,犊皮纸;喷墨打印/RIVES BFK printmaking paper light weight, PVC Sheet, vellum; inkjet print
2016年秋至2017年春;芝加哥,伊利诺伊州/Fall 2016–Spring 2017; Chicago, IL
在我小时候就开始学习绘画和钢琴,且我非常享受。因为我选择了视觉艺术作为我的专业,所以我一直想尝试用视觉艺术作为载体来呈现音乐。研究生毕业设计给了我很好的机会来探索这个领域。
巴赫的《平均律钢琴曲集》被誉为音乐届的 “旧约圣经”。当我在浏览整部乐集时,发现了一个非常有趣又神秘的现象:在不同曲子里有不同的图案发生重复构成。这一点激发了我对巴赫神秘的作曲公式的好奇心。
基于大量调研与试验,我找到了属于我的几何的、建筑型的、数学的并拥有严谨逻辑的视觉语言并用其把巴赫的《平均律钢琴曲集》转译成有趣且吸引人的立体结构,使观者能够更直观地理解他的音乐结构。(下图即巴赫《平均律钢琴曲集》中 “C大调前奏曲与赋格” 的前奏曲。从图中可以看到上升–下降–上升的图案贯穿全曲。)
I started to learn both painting and piano performing when I was a kid, and I love both of them. Since I chose visual art as my career, I always hold the thoughts to use visual arts as a form to visualize music. The M.F.A. Thesis is a perfect timing for me to dive into this area.
Bach’s “Well-Tempered Clavichord” is famous for being recognized as the “Old Testament” in music. When I was looking through the whole score sheets collection, I found out a very interesting and mysterious phenomenon that there are different patterns repeating within different pieces, which triggered my curiosity of Bach’s secret composing equation.
Based on numerous research and experimentation, I created my own geometric, architectural, mathematical, and logical visual language to translate Bach’s “Well-Tempered Clavichord” into appealing and seductive sculptural forms to offer a better and easier way to understand his music. (The image below is Bach’s manuscript of the first Prelude from his “Well-Tempered Clavichord,” whose ascending–descending–ascending pattern is happening all over the piece.)

芝加哥艺术学院2017届研究生毕业展/SAIC 2017 M.F.A. Show
2017年04月28日至05月16日/April 28th–May 16th, 2017
芝加哥艺术学院沙利文画廊/Sullivan Galleries, School of the Art Institute of Chicago









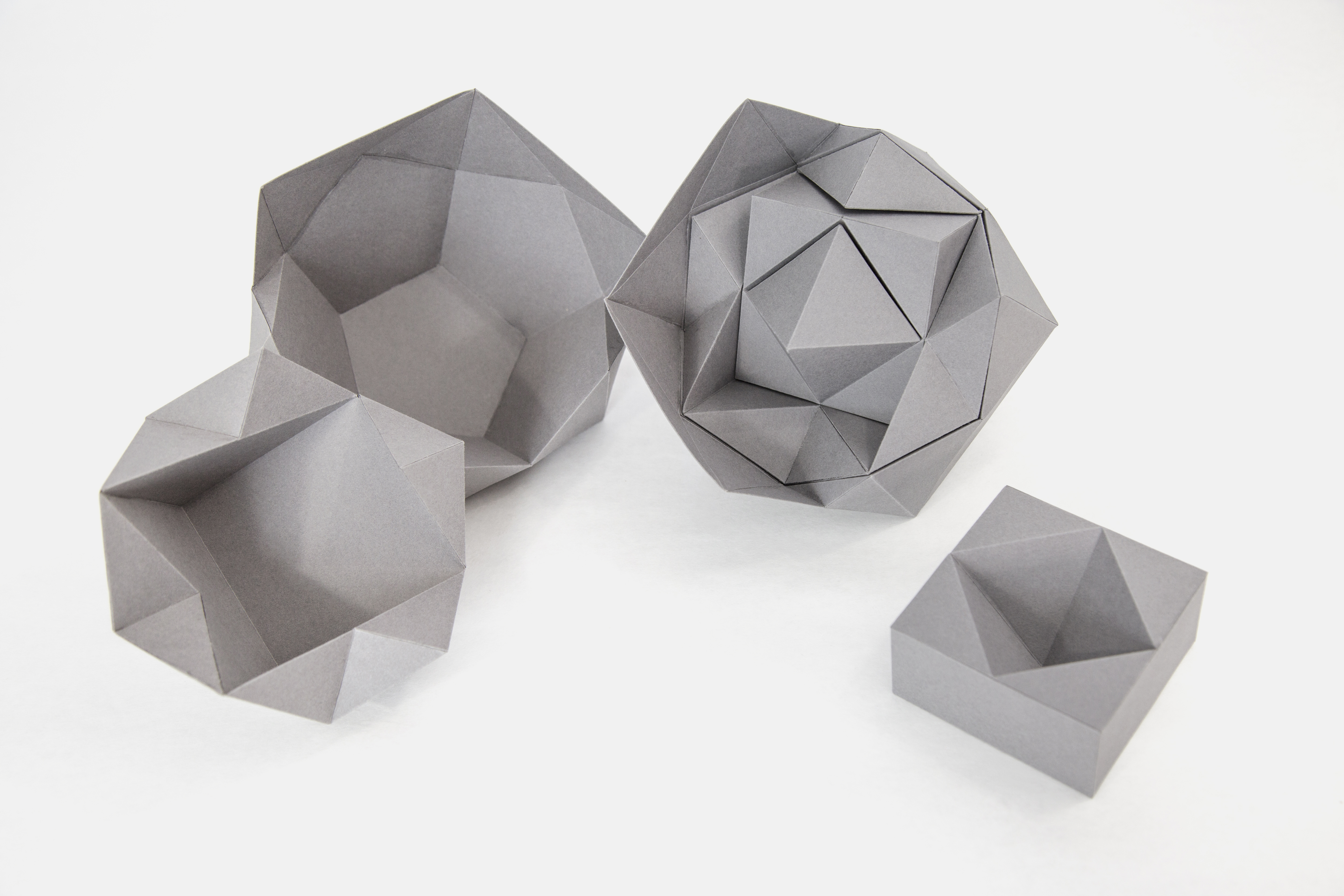
试验与运算/Experimentation & Calculation
简单模型中的数学运算相对容易,例如在正方体中嵌入正八面体。但我在最复杂的正二十面体中嵌入正十二面体结构上周旋了两个月之久。最终,我通过对它们的嵌套关系进行解剖,用实体与视觉的方法掌握了空间中一个单元的运作机制和多个单元的链接关系。下图即我所使用的解剖模型:
The math happening in the simple ones such as an octahedeon nesting in a cube is pretty easy to calculate. But I was stuck at one of the most intricate ones—a dodecahedron nesting in an icosahedron—for quite a long time, almost two months. Eventually, I did “antatomy” to this interrelationship using a physical and visual way to figure out how it spatially works on one single unit and how units are connected together. Here is the anatomical structure that I was using:


在解决空间与数学嵌套关系后,我把实验结果应用于巴赫赋格的层叠结构上来检验其可行性:将正八面体嵌套于正六面体/正方体内嵌套于正十二面体内嵌套于正二十面体内。
After figuring out the spatial and mathematic nesting relationship, I utilized what I found onto the layering structure of Bach’s Fugue to test it out—An Octahedron in a Hexahedron/Cube in a Dodecahedron in an Icosahedron.

探索与分析/Exploration & Analysing
基于大量试验与数学演算(主要为勾股定理与三角函数),每组聚氯乙烯立体都固体化与视觉化了巴赫《平均律钢琴曲集》中前奏曲与赋格相互联结、相互链锁、相互层叠并相互嵌套的结构关系。
Base on numerals experimentations and mathematical calculation (mainly Pythagorean Theorem and trigonometry), each one of the transparent sculptures solidifies and visualizes the intertwining, interlocking, layering, and nesting structures of the Preludes and Fugues from Bach’s “Well-Tempered Clavichord.”
1. 《平均律钢琴曲集》,48切面保罗 · 沙茨可展曲面/“Well-Tempered Clavichord,” 48-facet Oloid
保罗 · 沙茨可展曲面是一个三维立体的曲面几何形,于1929年由保罗 · 沙茨所发现。它是一个基于两个相互垂直的全等圆骨架而成的凸壳体,每个圆的圆心都恰好落在另一个圆的圆周且两圆的圆心距即圆的半径。圆周的1/3落在该凸壳体内,所以该立体型也可以被定义为由两个相互扭转了4π/3的两个等量弧形所形成的凸壳体。
保罗 · 沙茨可展曲面只有一个连续的外表面,类似更具体块感的莫比乌斯环,没有明确的开始与结束,整个形体就是一个整体,可以以任意一点作为起始点并在旋转一周后回到原点。巴赫的《平均律钢琴曲集》上下册共计48首前奏曲与赋格,所以我将保罗 · 沙茨可展曲面切割成48个切面来视觉化整部曲集的结构。所有的前奏曲与赋格切面相互串联形成了一个完整的类环状连续体,始于C大调回归C大调。该48切面可展曲面可以以任意切面为底面维持平衡状态,如同巴赫的《平均律钢琴曲集》,可以始于任意调性而回归本源。曲集的上下册拥有相同的调性编纂结构,它们相互模仿、相互支撑、相互维持,使整部曲集的更加牢固地锁定在一起形成了坚如磐石的稳定结构。
An oloid is a three-dimensional curved geometric object that was discovered by Paul Schatz in 1929. It is the convex hull of a skeletal frame made by placing two linked congruent circles in perpendicular planes, so that the center of each circle lies on the edge of the other circle. The distance between the circle centers equals the radius of the circles. One third of each circle's perimeter lies inside the convex hull, so the same shape may be also formed as the convex hull of the two remaining circular arcs each spanning an angle of 4π/3.
The Oloid has and only has one continuous surface. It is more like a cubified and solidified Mobius Strip, which has no starting and ending points and the entire form is a complete entity. It can start at any point and will travel back to the same one. Bach’s “Well-Tempered Clavichord” contains 48 Preludes and Fugues. Therefore, I cut Paul Schatz’s Oloid into 48 facets to visualize the structure of it. All the Preludes and Fugues are connected and following each other to compose an integrated looping architecture, starting from C major and go back to it. The 48-facet Oloid can stand on any single one of its facet, just like Bach’s Clavichord, can be considered starting from any tunality and going back to the same one. Its Volume I and Volume II share eaxctly the same compiling structure and they are mimicking, supporting, and backing up each other to shape a better locked and reinforced rock solid stability.
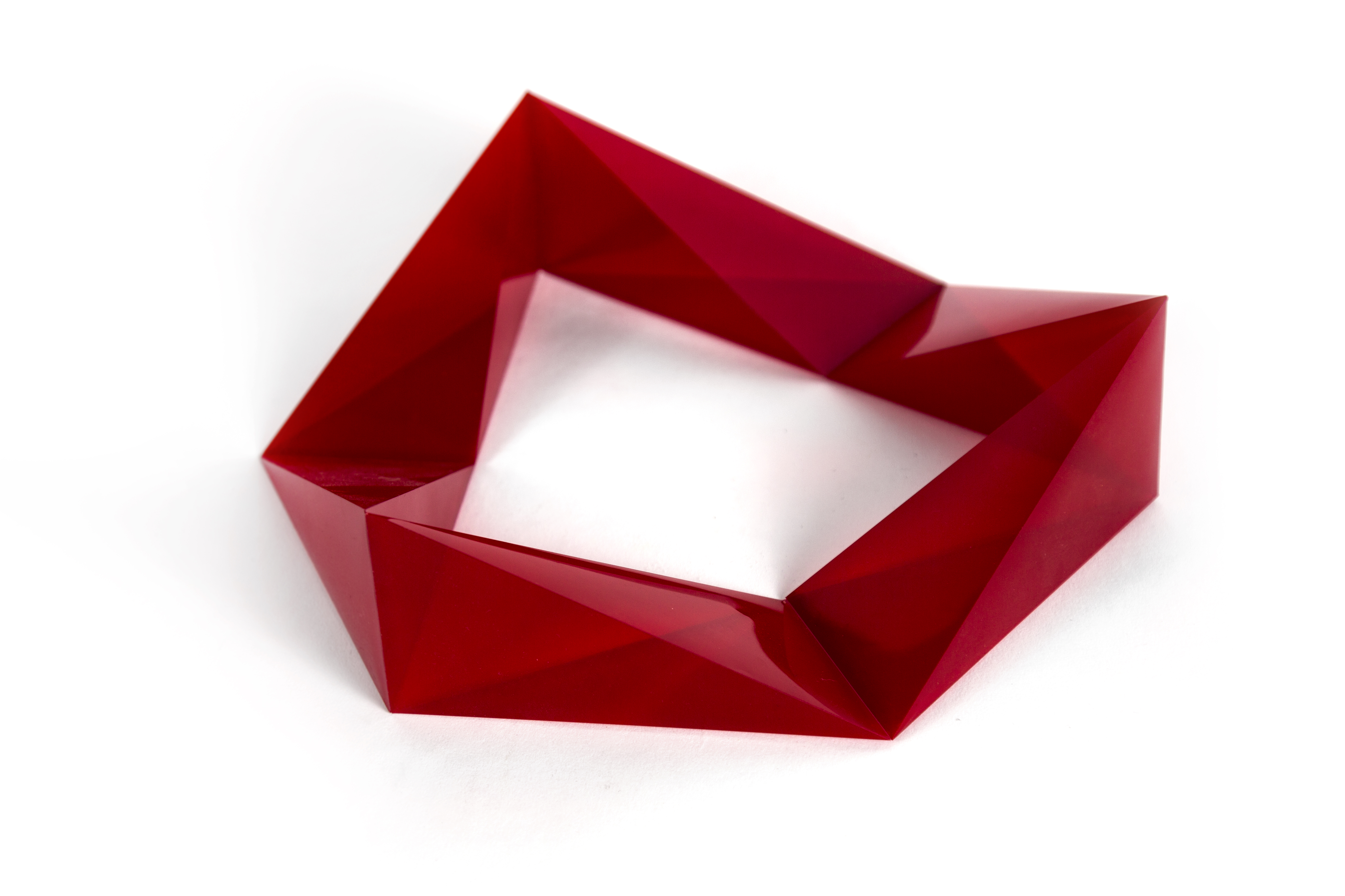
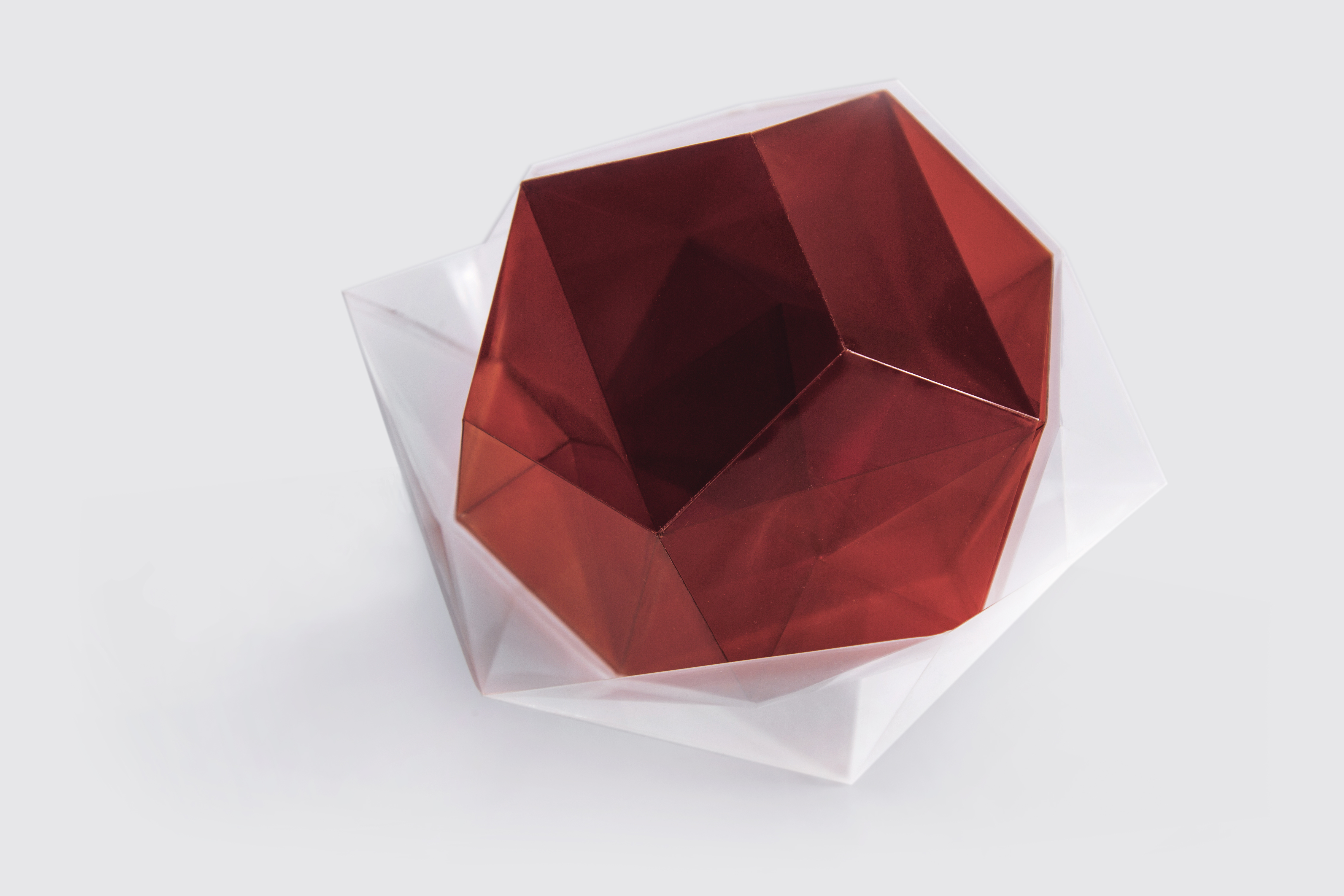
2. 上/下册,可逆转立方体内嵌于正十二面体/Volumes, Invertible Cube in a Dodecahedron
巴赫的《平均律钢琴曲集》上下册不光是含有24首独立的前奏曲与赋格的两本书。它们的调性完全遵循了从C大调、c小调一直上升到B大调、b小调的完整八度,最终还能回归到C大调从而形成一个完整的环形结构。下图是该环形结构的可视化结构。
The two volumes of Bach’s “Well-Tempered Clavichord” is more than just two books containing 24 separate pieces. The tonalities of them are in the exact arrangement of an octave from C major & C minor all to way to B major & B minor, which is followed by C major again to complete a loop. The one below is the visualization of the looping orchestration.
静态视图/Stationary Views:

整部曲集的可视化结构为可逆转立方体,它有两个榫头,被嵌入在正十二面体中。外层正十二面体为把整本曲集结构整合在一起的壳(qiào)。打开它后,即可分离可逆转立方体。其主体部分可持续向内或向外翻转并回到初始位置,和巴赫的《平均律钢琴曲集》调性为相同结构—始于立方体形态/C大调并回归原点。
The structural form is an Invertible Cube with two Keys being nested in a dodecahedron. The outside layer—dodecahedron—is the shield to embrace all the pieces as a whole. When it is being opened, the invertible cube can be revealed and the main body of the Invertible Cube can be twisted inward and outward and can go back to the original position repeatedly and continuously, which shares the same pattern as Bach’s “Well-Tempered Clavichord’s” tonalities—starting from the cube position/C major and ends at the same.
动态视图/Motional Views:

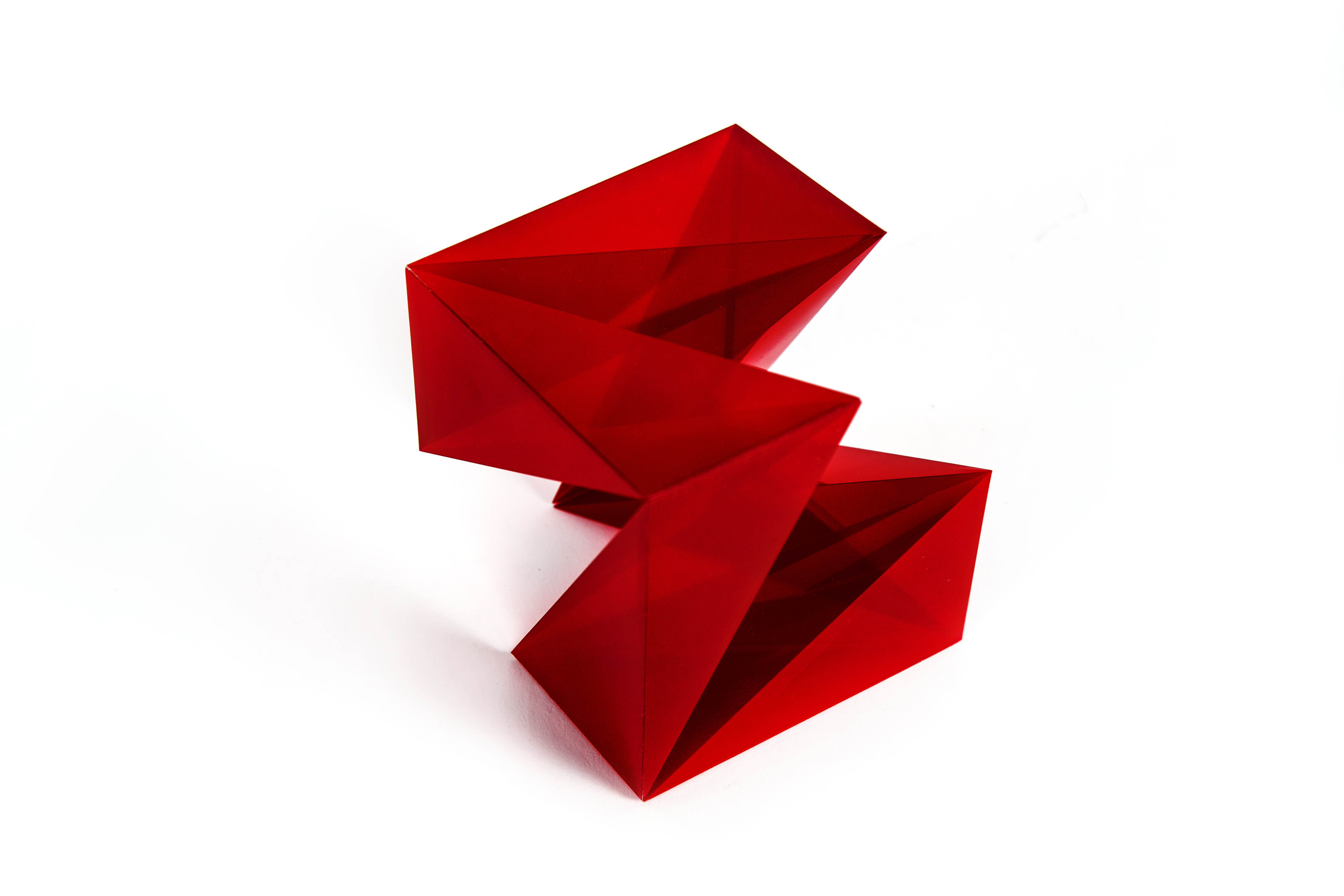




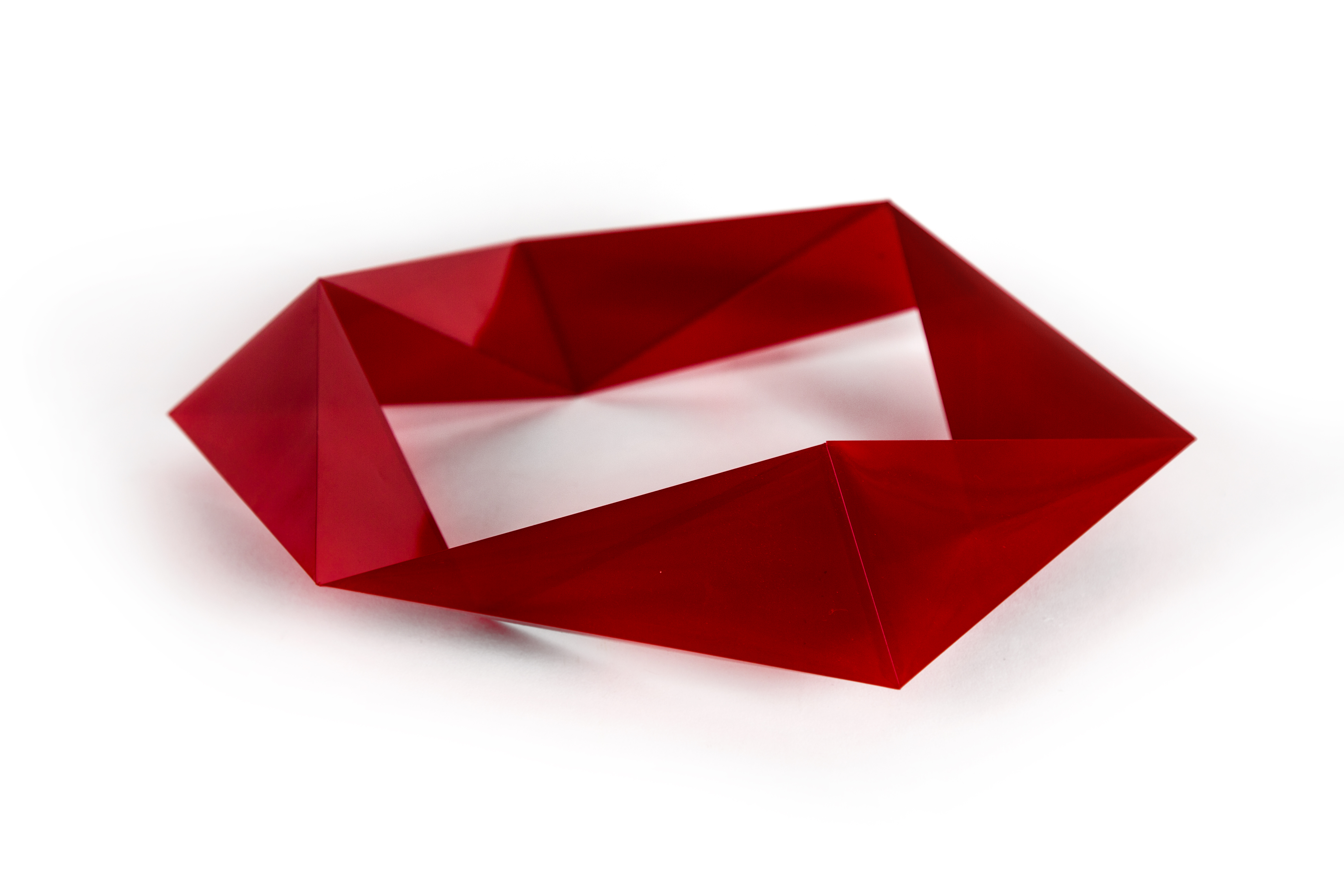

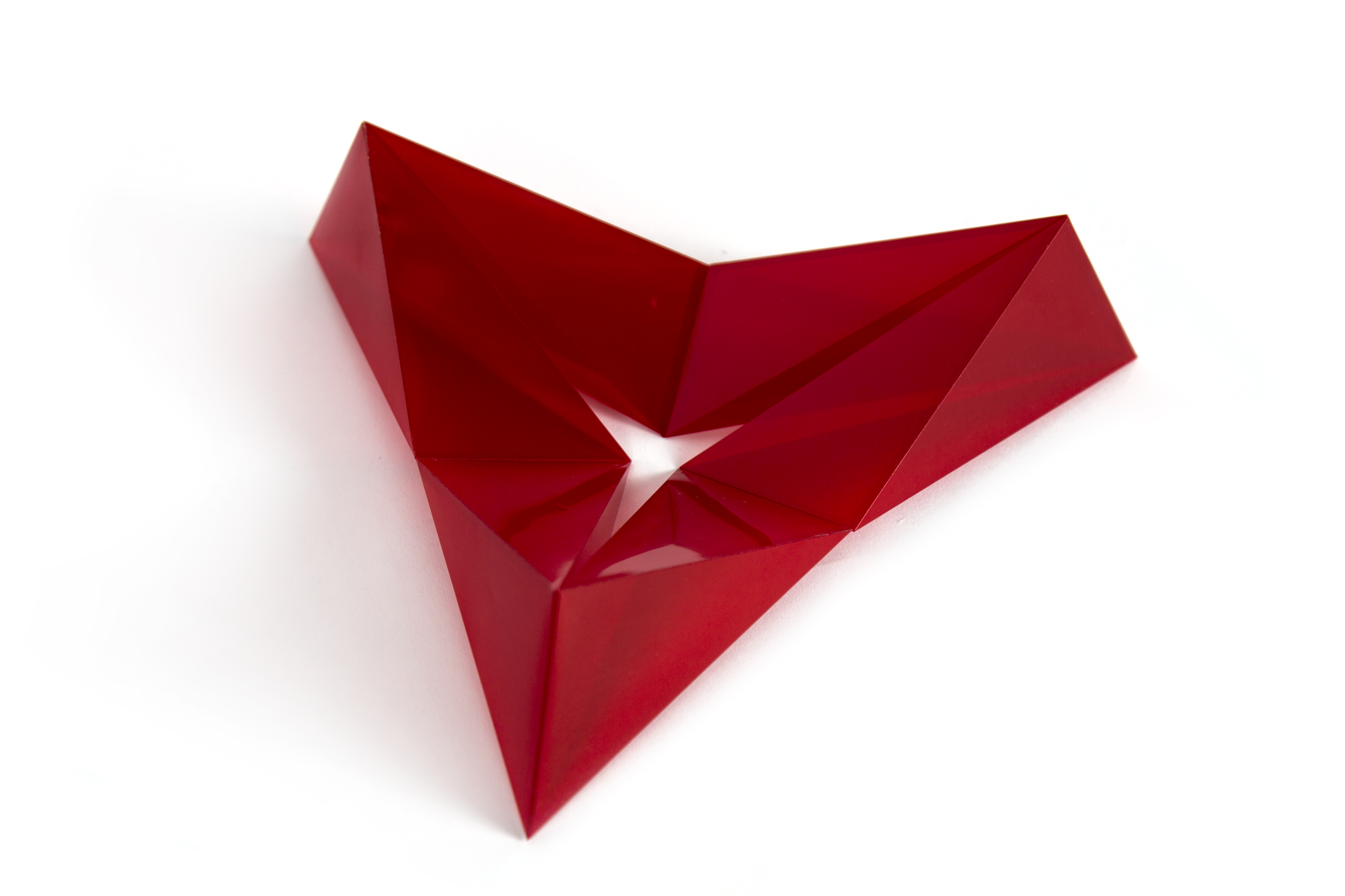


6. 赋格,层叠与嵌套结构/Fugue, Layering & Nesting Structure
正八面体内嵌于正六面体/正方体内嵌于正十二面体内嵌于正二十面体
An Octahedron in a Hexahedron/Cube in a Dodecahedron in an Icosahedron
《平均律钢琴曲集》中的赋格均为层叠与嵌套结构。每一首曲子都由 “主题” 串联,主题由 “动机” 构成,而动机中还包含 “动机中的动机”。这就和摩天大楼的建筑结构相同—表皮包裹着室内空间,室内空间由骸骨支撑,而骸骨受最基本的建筑功能所驱动。根据这套逻辑,赋格被理解为是拥有建筑机构的、雕塑形态的和数学逻辑的。因此,将音乐结构与几何形体重构实现了对赋格结构的视觉化。
Fugues from the “Well-Tempered Clavichord” is of layering and nesting structure. Each piece is composed of “themes” composed of “motifs” composed of “motif in motifs,” which is literally the same as the architectural structure of an edifice—the facades out of interior spaces out of skeletons out of constructional functionalities. Due to this logic, the fugues are considered architectural, sculptural, and mathematical. Therefore, the mapping of the musical and geometric structure realizes the visualization.
正二十面体—整首赋格/Icosahedron—the Whole Fugue
正十二面体—主题/Dodecahedron—Theme
正六面体(正方体)—动机/Hexahedron (Cube)—Motif
正八面体—动机中的动机/Octahedron—Motif in Motif


毕业设计导师的评价/Comments from My Thesis Advisor
from: 史蒂芬 · 法雷尔/Stephen Farrell <sfarre@saic.edu>
to: Li Han <lhan4@saic.edu>
date: Mon, Apr 24, 2017 at 12:49 PM
subject: 回复:毕业展最终更新,嘻嘻/RE: The Final Updating about the Show, Lol
“太惊艳了,韩立。这套毕设简直是不可思议、引人入胜、高智商、‘高级’ 且 ‘高端’。你在毕设的整个过程中始终保持了高质量和探究思维。我们对你的完成成果感到非常骄傲。你在整个毕业展中代表了视觉传达系的精神。在你的毕业设计中你通过一种全新的视觉语言映射了巴赫。”
现在你终于可以放松下来开始享受了!
这周见,
史蒂芬
“Many many wows, Li. It’s fantastic, mesmerizing, intellectual, ‘fancy,’ AND ‘high-end.’ You maintained quality and the thread of inquiry through the entire process, right up until the end—we are all very proud of what you’ve accomplished and that you are representing Visual Communication Department in the show. You are onto something here in a visual language that speaks Bach in a whole new way.”
Now you can relax and enjoy!
See you this week,
Stephen

视觉传达设计系,副教授/Associate Professor, Department of Visual Communication Design
芝加哥艺术学院/The School of the Art Institute of Chicago
37南沃巴什大道,房间1101-C/37 South Wabash Avenue, rm 1101-C
芝加哥 60603 美国/Chicago 60603 USA
电话/tel. 312.629.1672
邮箱/eml. sfarre@saic.edu
Special Thanks to
史蒂芬 · 法雷尔/Stephen Farrell雅各布 · 里斯托/Jacob Ristau
闫 大卫 [Dawei (David) Yan]
丹尼尔 · 摩根泰勒/Daniel Morgenthaler
肖恩 · 德克尔/Shawn Decker
玄 明娥/Myungah Hyon (현 명아)
郭 烨 (Ye Guo)
陈 思 (Si Chen)
李 劭恆 (Shau Heng Li)
#音乐可视化/music visualization
#学科交叉/interdisciplinary
#纸雕塑/paper sculpture
#数学/mathematics
#艺术家书/artist’s book
#自主探究项目/self-generated project